Anamorphic Prism Pairs

- Transform Elliptical Laser Diode Beams Into Nearly Circular Beams
- Magnification from 2.0 to 4.0
- Three Broadband AR Coating Choices
PS879-A
Mounted, Pre-Aligned Prism Pair
SM05
Threading
PS871-B
Unmounted Prism Pair

Please Wait
| General Specifications | |
|---|---|
| Material | N-SF11a (Uncoated, -B, -C Coating) N-KZFS8a (Uncoated, -A Coating) |
| Dimensional Tolerances |
Unmounted: ±0.15 mm |
| Angular Tolerances |
Unmounted: ±15 arcmin |
| Maximum Input Beam Size |
Unmounted: >Ø8.0 mm Mounted: 5.1 mm Minor Axis, >Ø8.0 mm Major Axis |
| Maximum Output Beam Size |
>Ø8.0 mm |
| Surface Flatness | λ/8 @ 633.8 nm (Unmounted) λ/10 @ 632.8 nm (Mounted) |
| Surface Quality | 40-20 Scratch-Dig |
| Coating Options | Uncoated AR Coated: 350 - 700 nm (-A) AR Coated: 650 - 1050 nm (-B) AR Coated: 1050 - 1700 nm (-C) |
| Material Damage Threshold |
N-KZFS8 -A Coating: 5 J/cm2 (532 nm, 10 ns, 10 Hz, Ø0.456 mm) N-SF11 -B Coating: 10 J/cm2 (1064 nm, 10 ns, 10 Hz, Ø0.454 mm) N-SF11 -C Coating: 10 J/cm2 (1064 nm, 10 ns, 10 Hz, Ø0.521 mm) |
Features
- Change Ellipticity of Beam Shape to Form Circular or Elliptical Output
- Pairs Available Unmounted or Mounted in Ø1" Housings
- Magnifications from 2.0 to 4.0
- Uncoated or AR Coated for 350 - 700 nm, 650 - 1050 nm, or 1050 - 1700 nm
Thorlabs' Anamorphic Prism Pairs are used to transform elliptical laser diode beams into nearly circular beams by magnifying the elliptical beam in one dimension. They can also be used to convert a circular beam into an elliptical beam. Available unmounted or mounted in Ø1" housings that feature SM05 (0.535"-40) threads on one end, these prism pairs can be purchased uncoated (unmounted only) or with an antireflection (AR) coating for the 350 - 700 nm, 650 - 1050 nm, or 1050 - 1700 nm spectral ranges. Mounted prism pairs can be chosen with magnifications from 2.0 to 4.0.
An average throughput of 95% can be achieved if the prisms are oriented such that the incident light enters the prism pair at Brewster's angle and each surface has the appropriate AR coating for the wavelength of the incident light. Our mounted prisms are manufactured to allow light to be incident at the Brewster angle, and therefore, only our unmounted prisms will need to be manually oriented to achieve the aforementioned average throughput. Furthermore, to minimize back reflections, p-polarized light should be incident on the prisms when used in the configuration shown below. Please note that clear aperture is >Ø8.0 mm. For the mounted prisms, the input beam height may be limited by the entrance opening height of 5.1 mm.
Beam shaping can also be accomplished by using cylindrical lenses, which provide one-dimensional shaping of a beam. For more information about Thorlabs' extensive line of prisms, please refer to the Prism Guide tab above.
Click to Enlarge
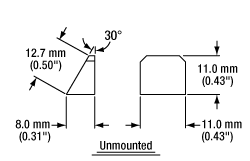
Unmounted Prism Mechanical Drawing
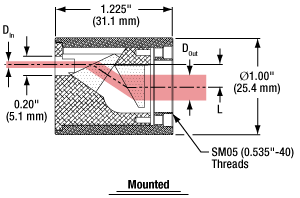
Click for Details
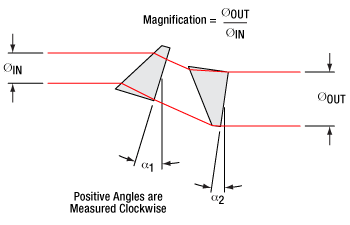
L is the displacement between the input and output optical beam axis and is given in the tables below. Please note that the output beam will not exit at the center of the aperture. Magnification = DOut / DIn
N-KZFS8 Reflectance and Transmission
N-SF11 Reflectance and Transmission
The graphs below show the magnification factor as a function of wavelength for all of our mounted anamorphic prism pairs.
Anamorphic Prism Pair Dependence on Angle

The plots below show the angles at which the prisms must be set for various magnifications:
Click to Enlarge
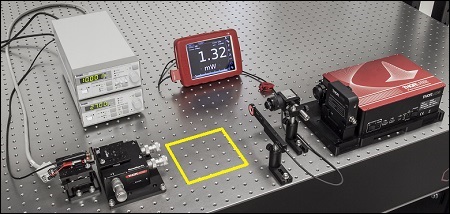
The beam circularization systems were placed in the area of the experimental setup highlighted by the yellow rectangle.

Click to Enlarge
Spatial Filter System

Click to Enlarge
Anamorphic Prism Pair System

Click to Enlarge
Cylindrical Lens Pair System
Comparison of Circularization Techniques for Elliptical Beams
Edge-emitting laser diodes emit elliptical beams as a consequence of the rectangular cross sections of their emission apertures. The component of the beam corresponding to the narrower dimension of the aperture has a greater divergence angle than the orthogonal beam component. As one component diverges more rapidly than the other, the beam shape is elliptical rather than circular.
Elliptical beam shapes can be undesirable, as the spot size of the focused beam is larger than if the beam were circular, and larger spot sizes have lower irradiances (power per area). Several different techniques can be used to circularize an elliptical beam, and we experimented with and compared the performance of three methods based on a pair of cylindrical lenses, an anamorphic prism pair, and a spatial filter. The characteristics of the circularized beams were evaluated by performing M2 measurements, wavefront measurements, and measuring the transmitted power.
While we demonstrated that each circularization technique improves the circularity of the elliptical input beam, we showed that each technique provides a different balance of circularization, beam quality, and transmitted power. Our results, which are documented in this Lab Fact, indicate that an application's specific requirements will determine which is the best circularization technique to choose.
Experimental Design and Setup
The experimental setup is shown in the picture at the top-right. The elliptically-shaped, collimated beam of a temperature-stabilized 670 nm laser diode was input to each of our circularization systems. Collimation results in a low-divergence beam, but it does not affect the beam shape.
The beam circularization systems, shown to the right, were placed, one at a time, in the vacant spot in the setup highlighted by the yellow rectangle. With this arrangement, it was possible to use the same experimental conditions when evaluating each circularization technique, which allowed the performance of each to be directly compared with the others. Some information describing selection and configuration procedures for several components used in this experimental work can be accessed by clicking the following hyperlinks:
- Mounting Laser Diodes
- Driving a Laser Diode
- Selecting a Collimating Lens
- Aspheric Lenses
- Spatial Filters
The characteristics of the beams output by the different circularization systems were evaluated by making measurements using a power meter, a wavefront sensor, and an M2 system. In the image of the experimental setup, all of these systems are shown on the right side of the table for illustrative purposes; they were used one at a time. The power meter was used to determine how much the beam circularization system attenuated the intensity of the input laser beam. The wavefront sensor provided a way to measure the abberations of the output beam. The M2 system measurement describes the resemblence of the output beam to a Gaussian beam. Ideally, the circularization systems would not attenuate or abberate the laser beam, and they would output a perfectly Gaussian beam.
Edge-emitting laser diodes also emit astigmatic beams, and it can be desirable to force the displaced focal points of the orthogonal beam components to overlap. Of the three circularization techniques investigated in this work, only the cylindrical lens pair can also compensate for astigmatism. The displacement between the focal spots of the orthogonal beam components were measured for each circularization technique. In the case of the cylindrical lens pair, their configuration was tuned to minimize the astigmatism in the laser beam. The astigmatism was reported as a normalized quantity.
Experimental Results
The experimental results are summarized in the following table, in which the green cells identify the best result in each category. Each circularization approach has its benefits. The best circularization technique for an application is determined by the system’s requirements for beam quality, transmitted optical power, and setup constraints.
Spatial filtering significantly improved the circularity and quality of the beam, but the beam had low transmitted power. The cylindrical lens pair provided a well-circularized beam and balanced circularization and beam quality with transmitted power. In addition, the cylindrical lens pair compensated for much of the beam's astigmatism. The circularity of the beam provided by the anamorphic prism pair compared well to that of the cylindrical lens pair. The beam output from the prisms had better M2 values and less wavefront error than the cylindrical lenses, but the transmitted power was lower.
| Method | Beam Intensity Profile | Circularitya | M2 Values | RMS Wavefront | Transmitted Power | Normalized Astigmatismb |
|---|---|---|---|---|---|---|
| Collimated Source Output (No Circularization Technique) |
 Click to Enlarge Scale in Microns |
0.36 | Y Axis: 1.63 |
0.17 | Not Applicable | 0.67 |
| Cylindrical Lens Pair |  Click to Enlarge Scale in Microns |
0.84 | X Axis: 1.90 Y Axis: 1.93 |
0.30 | 91% | 0.06 |
| Anamorphic Prism Pair |  Click to Enlarge Scale in Microns |
0.82 | X Axis: 1.60 Y Axis: 1.46 |
0.16 | 80% | 1.25 |
| Spatial Filter |  Click to Enlarge Scale in Microns |
0.93 | X Axis: 1.05 Y Axis: 1.10 |
0.10 | 34% | 0.36 |
Components used in each circularization system were chosen to allow the same experimental setup be used for all experiments. This had the desired effect of allowing the results of all circularization techniques to be directly compared; however, optimizing the setup for a circularization technique could have improved its performance. The mounts used for the collimating lens and the anamorphic prism pair enabled easy manipulation and integration into this experimental system. It is possible that using smaller mounts would improve results by allowing the members of each pair to be more precisely positioned with respect to one another. In addition, using made-to-order cylindrical lenses with customized focal lengths may have improved the results of the cylindrical lens pair circularization system. All results may have been affected by the use of the beam profiler software algorithm to determine the beam radii used in the circularity calculation.
| Damage Threshold Specifications | ||
|---|---|---|
| Coating Designation (Item # Suffix) |
Substrate Material |
Damage Threshold |
| -A | N-KZFS8 | 5 J/cm2 (532 nm, 10 ns, 10 Hz, Ø0.456 mm) |
| -B | N-SF11 | 10 J/cm2 (1064 nm, 10 ns, 10 Hz, Ø0.454 mm) |
| -C | N-SF11 | 10 J/cm2 (1064 nm, 10 ns, 10 Hz, Ø0.521 mm) |
Damage Threshold Data for Thorlabs' Anamorphic Prism Pairs
The specifications to the right are measured data for Thorlabs' anamorphic prism pairs. Damage threshold specifications are constant for a given coating, regardless of the magnification or mounting option of the prism.
Laser Induced Damage Threshold Tutorial
The following is a general overview of how laser induced damage thresholds are measured and how the values may be utilized in determining the appropriateness of an optic for a given application. When choosing optics, it is important to understand the Laser Induced Damage Threshold (LIDT) of the optics being used. The LIDT for an optic greatly depends on the type of laser you are using. Continuous wave (CW) lasers typically cause damage from thermal effects (absorption either in the coating or in the substrate). Pulsed lasers, on the other hand, often strip electrons from the lattice structure of an optic before causing thermal damage. Note that the guideline presented here assumes room temperature operation and optics in new condition (i.e., within scratch-dig spec, surface free of contamination, etc.). Because dust or other particles on the surface of an optic can cause damage at lower thresholds, we recommend keeping surfaces clean and free of debris. For more information on cleaning optics, please see our Optics Cleaning tutorial.
Testing Method
Thorlabs' LIDT testing is done in compliance with ISO/DIS 11254 and ISO 21254 specifications.
First, a low-power/energy beam is directed to the optic under test. The optic is exposed in 10 locations to this laser beam for 30 seconds (CW) or for a number of pulses (pulse repetition frequency specified). After exposure, the optic is examined by a microscope (~100X magnification) for any visible damage. The number of locations that are damaged at a particular power/energy level is recorded. Next, the power/energy is either increased or decreased and the optic is exposed at 10 new locations. This process is repeated until damage is observed. The damage threshold is then assigned to be the highest power/energy that the optic can withstand without causing damage. A histogram such as that below represents the testing of one BB1-E02 mirror.

The photograph above is a protected aluminum-coated mirror after LIDT testing. In this particular test, it handled 0.43 J/cm2 (1064 nm, 10 ns pulse, 10 Hz, Ø1.000 mm) before damage.
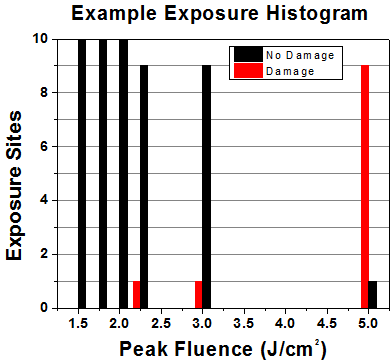
| Example Test Data | |||
|---|---|---|---|
| Fluence | # of Tested Locations | Locations with Damage | Locations Without Damage |
| 1.50 J/cm2 | 10 | 0 | 10 |
| 1.75 J/cm2 | 10 | 0 | 10 |
| 2.00 J/cm2 | 10 | 0 | 10 |
| 2.25 J/cm2 | 10 | 1 | 9 |
| 3.00 J/cm2 | 10 | 1 | 9 |
| 5.00 J/cm2 | 10 | 9 | 1 |
According to the test, the damage threshold of the mirror was 2.00 J/cm2 (532 nm, 10 ns pulse, 10 Hz, Ø0.803 mm). Please keep in mind that these tests are performed on clean optics, as dirt and contamination can significantly lower the damage threshold of a component. While the test results are only representative of one coating run, Thorlabs specifies damage threshold values that account for coating variances.
Continuous Wave and Long-Pulse Lasers
When an optic is damaged by a continuous wave (CW) laser, it is usually due to the melting of the surface as a result of absorbing the laser's energy or damage to the optical coating (antireflection) [1]. Pulsed lasers with pulse lengths longer than 1 µs can be treated as CW lasers for LIDT discussions.
When pulse lengths are between 1 ns and 1 µs, laser-induced damage can occur either because of absorption or a dielectric breakdown (therefore, a user must check both CW and pulsed LIDT). Absorption is either due to an intrinsic property of the optic or due to surface irregularities; thus LIDT values are only valid for optics meeting or exceeding the surface quality specifications given by a manufacturer. While many optics can handle high power CW lasers, cemented (e.g., achromatic doublets) or highly absorptive (e.g., ND filters) optics tend to have lower CW damage thresholds. These lower thresholds are due to absorption or scattering in the cement or metal coating.

LIDT in linear power density vs. pulse length and spot size. For long pulses to CW, linear power density becomes a constant with spot size. This graph was obtained from [1].
Pulsed lasers with high pulse repetition frequencies (PRF) may behave similarly to CW beams. Unfortunately, this is highly dependent on factors such as absorption and thermal diffusivity, so there is no reliable method for determining when a high PRF laser will damage an optic due to thermal effects. For beams with a high PRF both the average and peak powers must be compared to the equivalent CW power. Additionally, for highly transparent materials, there is little to no drop in the LIDT with increasing PRF.
In order to use the specified CW damage threshold of an optic, it is necessary to know the following:
- Wavelength of your laser
- Beam diameter of your beam (1/e2)
- Approximate intensity profile of your beam (e.g., Gaussian)
- Linear power density of your beam (total power divided by 1/e2 beam diameter)
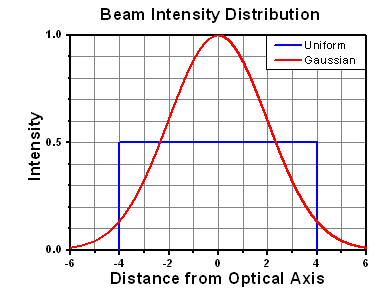
Thorlabs expresses LIDT for CW lasers as a linear power density measured in W/cm. In this regime, the LIDT given as a linear power density can be applied to any beam diameter; one does not need to compute an adjusted LIDT to adjust for changes in spot size, as demonstrated by the graph to the right. Average linear power density can be calculated using the equation below.

The calculation above assumes a uniform beam intensity profile. You must now consider hotspots in the beam or other non-uniform intensity profiles and roughly calculate a maximum power density. For reference, a Gaussian beam typically has a maximum power density that is twice that of the uniform beam (see lower right).
Now compare the maximum power density to that which is specified as the LIDT for the optic. If the optic was tested at a wavelength other than your operating wavelength, the damage threshold must be scaled appropriately. A good rule of thumb is that the damage threshold has a linear relationship with wavelength such that as you move to shorter wavelengths, the damage threshold decreases (i.e., a LIDT of 10 W/cm at 1310 nm scales to 5 W/cm at 655 nm):

While this rule of thumb provides a general trend, it is not a quantitative analysis of LIDT vs wavelength. In CW applications, for instance, damage scales more strongly with absorption in the coating and substrate, which does not necessarily scale well with wavelength. While the above procedure provides a good rule of thumb for LIDT values, please contact Tech Support if your wavelength is different from the specified LIDT wavelength. If your power density is less than the adjusted LIDT of the optic, then the optic should work for your application.
Please note that we have a buffer built in between the specified damage thresholds online and the tests which we have done, which accommodates variation between batches. Upon request, we can provide individual test information and a testing certificate. The damage analysis will be carried out on a similar optic (customer's optic will not be damaged). Testing may result in additional costs or lead times. Contact Tech Support for more information.
Pulsed Lasers
As previously stated, pulsed lasers typically induce a different type of damage to the optic than CW lasers. Pulsed lasers often do not heat the optic enough to damage it; instead, pulsed lasers produce strong electric fields capable of inducing dielectric breakdown in the material. Unfortunately, it can be very difficult to compare the LIDT specification of an optic to your laser. There are multiple regimes in which a pulsed laser can damage an optic and this is based on the laser's pulse length. The highlighted columns in the table below outline the relevant pulse lengths for our specified LIDT values.
Pulses shorter than 10-9 s cannot be compared to our specified LIDT values with much reliability. In this ultra-short-pulse regime various mechanics, such as multiphoton-avalanche ionization, take over as the predominate damage mechanism [2]. In contrast, pulses between 10-7 s and 10-4 s may cause damage to an optic either because of dielectric breakdown or thermal effects. This means that both CW and pulsed damage thresholds must be compared to the laser beam to determine whether the optic is suitable for your application.
| Pulse Duration | t < 10-9 s | 10-9 < t < 10-7 s | 10-7 < t < 10-4 s | t > 10-4 s |
|---|---|---|---|---|
| Damage Mechanism | Avalanche Ionization | Dielectric Breakdown | Dielectric Breakdown or Thermal | Thermal |
| Relevant Damage Specification | No Comparison (See Above) | Pulsed | Pulsed and CW | CW |
When comparing an LIDT specified for a pulsed laser to your laser, it is essential to know the following:

LIDT in energy density vs. pulse length and spot size. For short pulses, energy density becomes a constant with spot size. This graph was obtained from [1].
- Wavelength of your laser
- Energy density of your beam (total energy divided by 1/e2 area)
- Pulse length of your laser
- Pulse repetition frequency (prf) of your laser
- Beam diameter of your laser (1/e2 )
- Approximate intensity profile of your beam (e.g., Gaussian)
The energy density of your beam should be calculated in terms of J/cm2. The graph to the right shows why expressing the LIDT as an energy density provides the best metric for short pulse sources. In this regime, the LIDT given as an energy density can be applied to any beam diameter; one does not need to compute an adjusted LIDT to adjust for changes in spot size. This calculation assumes a uniform beam intensity profile. You must now adjust this energy density to account for hotspots or other nonuniform intensity profiles and roughly calculate a maximum energy density. For reference a Gaussian beam typically has a maximum energy density that is twice that of the 1/e2 beam.
Now compare the maximum energy density to that which is specified as the LIDT for the optic. If the optic was tested at a wavelength other than your operating wavelength, the damage threshold must be scaled appropriately [3]. A good rule of thumb is that the damage threshold has an inverse square root relationship with wavelength such that as you move to shorter wavelengths, the damage threshold decreases (i.e., a LIDT of 1 J/cm2 at 1064 nm scales to 0.7 J/cm2 at 532 nm):

You now have a wavelength-adjusted energy density, which you will use in the following step.
Beam diameter is also important to know when comparing damage thresholds. While the LIDT, when expressed in units of J/cm², scales independently of spot size; large beam sizes are more likely to illuminate a larger number of defects which can lead to greater variances in the LIDT [4]. For data presented here, a <1 mm beam size was used to measure the LIDT. For beams sizes greater than 5 mm, the LIDT (J/cm2) will not scale independently of beam diameter due to the larger size beam exposing more defects.
The pulse length must now be compensated for. The longer the pulse duration, the more energy the optic can handle. For pulse widths between 1 - 100 ns, an approximation is as follows:

Use this formula to calculate the Adjusted LIDT for an optic based on your pulse length. If your maximum energy density is less than this adjusted LIDT maximum energy density, then the optic should be suitable for your application. Keep in mind that this calculation is only used for pulses between 10-9 s and 10-7 s. For pulses between 10-7 s and 10-4 s, the CW LIDT must also be checked before deeming the optic appropriate for your application.
Please note that we have a buffer built in between the specified damage thresholds online and the tests which we have done, which accommodates variation between batches. Upon request, we can provide individual test information and a testing certificate. Contact Tech Support for more information.
[1] R. M. Wood, Optics and Laser Tech. 29, 517 (1998).
[2] Roger M. Wood, Laser-Induced Damage of Optical Materials (Institute of Physics Publishing, Philadelphia, PA, 2003).
[3] C. W. Carr et al., Phys. Rev. Lett. 91, 127402 (2003).
[4] N. Bloembergen, Appl. Opt. 12, 661 (1973).
In order to illustrate the process of determining whether a given laser system will damage an optic, a number of example calculations of laser induced damage threshold are given below. For assistance with performing similar calculations, we provide a spreadsheet calculator that can be downloaded by clicking the button to the right. To use the calculator, enter the specified LIDT value of the optic under consideration and the relevant parameters of your laser system in the green boxes. The spreadsheet will then calculate a linear power density for CW and pulsed systems, as well as an energy density value for pulsed systems. These values are used to calculate adjusted, scaled LIDT values for the optics based on accepted scaling laws. This calculator assumes a Gaussian beam profile, so a correction factor must be introduced for other beam shapes (uniform, etc.). The LIDT scaling laws are determined from empirical relationships; their accuracy is not guaranteed. Remember that absorption by optics or coatings can significantly reduce LIDT in some spectral regions. These LIDT values are not valid for ultrashort pulses less than one nanosecond in duration.

A Gaussian beam profile has about twice the maximum intensity of a uniform beam profile.
CW Laser Example
Suppose that a CW laser system at 1319 nm produces a 0.5 W Gaussian beam that has a 1/e2 diameter of 10 mm. A naive calculation of the average linear power density of this beam would yield a value of 0.5 W/cm, given by the total power divided by the beam diameter:

However, the maximum power density of a Gaussian beam is about twice the maximum power density of a uniform beam, as shown in the graph to the right. Therefore, a more accurate determination of the maximum linear power density of the system is 1 W/cm.
An AC127-030-C achromatic doublet lens has a specified CW LIDT of 350 W/cm, as tested at 1550 nm. CW damage threshold values typically scale directly with the wavelength of the laser source, so this yields an adjusted LIDT value:

The adjusted LIDT value of 350 W/cm x (1319 nm / 1550 nm) = 298 W/cm is significantly higher than the calculated maximum linear power density of the laser system, so it would be safe to use this doublet lens for this application.
Pulsed Nanosecond Laser Example: Scaling for Different Pulse Durations
Suppose that a pulsed Nd:YAG laser system is frequency tripled to produce a 10 Hz output, consisting of 2 ns output pulses at 355 nm, each with 1 J of energy, in a Gaussian beam with a 1.9 cm beam diameter (1/e2). The average energy density of each pulse is found by dividing the pulse energy by the beam area:

As described above, the maximum energy density of a Gaussian beam is about twice the average energy density. So, the maximum energy density of this beam is ~0.7 J/cm2.
The energy density of the beam can be compared to the LIDT values of 1 J/cm2 and 3.5 J/cm2 for a BB1-E01 broadband dielectric mirror and an NB1-K08 Nd:YAG laser line mirror, respectively. Both of these LIDT values, while measured at 355 nm, were determined with a 10 ns pulsed laser at 10 Hz. Therefore, an adjustment must be applied for the shorter pulse duration of the system under consideration. As described on the previous tab, LIDT values in the nanosecond pulse regime scale with the square root of the laser pulse duration:

This adjustment factor results in LIDT values of 0.45 J/cm2 for the BB1-E01 broadband mirror and 1.6 J/cm2 for the Nd:YAG laser line mirror, which are to be compared with the 0.7 J/cm2 maximum energy density of the beam. While the broadband mirror would likely be damaged by the laser, the more specialized laser line mirror is appropriate for use with this system.
Pulsed Nanosecond Laser Example: Scaling for Different Wavelengths
Suppose that a pulsed laser system emits 10 ns pulses at 2.5 Hz, each with 100 mJ of energy at 1064 nm in a 16 mm diameter beam (1/e2) that must be attenuated with a neutral density filter. For a Gaussian output, these specifications result in a maximum energy density of 0.1 J/cm2. The damage threshold of an NDUV10A Ø25 mm, OD 1.0, reflective neutral density filter is 0.05 J/cm2 for 10 ns pulses at 355 nm, while the damage threshold of the similar NE10A absorptive filter is 10 J/cm2 for 10 ns pulses at 532 nm. As described on the previous tab, the LIDT value of an optic scales with the square root of the wavelength in the nanosecond pulse regime:

This scaling gives adjusted LIDT values of 0.08 J/cm2 for the reflective filter and 14 J/cm2 for the absorptive filter. In this case, the absorptive filter is the best choice in order to avoid optical damage.
Pulsed Microsecond Laser Example
Consider a laser system that produces 1 µs pulses, each containing 150 µJ of energy at a repetition rate of 50 kHz, resulting in a relatively high duty cycle of 5%. This system falls somewhere between the regimes of CW and pulsed laser induced damage, and could potentially damage an optic by mechanisms associated with either regime. As a result, both CW and pulsed LIDT values must be compared to the properties of the laser system to ensure safe operation.
If this relatively long-pulse laser emits a Gaussian 12.7 mm diameter beam (1/e2) at 980 nm, then the resulting output has a linear power density of 5.9 W/cm and an energy density of 1.2 x 10-4 J/cm2 per pulse. This can be compared to the LIDT values for a WPQ10E-980 polymer zero-order quarter-wave plate, which are 5 W/cm for CW radiation at 810 nm and 5 J/cm2 for a 10 ns pulse at 810 nm. As before, the CW LIDT of the optic scales linearly with the laser wavelength, resulting in an adjusted CW value of 6 W/cm at 980 nm. On the other hand, the pulsed LIDT scales with the square root of the laser wavelength and the square root of the pulse duration, resulting in an adjusted value of 55 J/cm2 for a 1 µs pulse at 980 nm. The pulsed LIDT of the optic is significantly greater than the energy density of the laser pulse, so individual pulses will not damage the wave plate. However, the large average linear power density of the laser system may cause thermal damage to the optic, much like a high-power CW beam.
Choosing a Collimation Lens for Your Laser Diode
Since the output of a laser diode is highly divergent, collimating optics are necessary. Aspheric lenses do not introduce spherical aberration and therefore are commonly chosen when the collimated laser beam is to be between one and five millimeters. A simple example will illustrate the key specifications to consider when choosing the correct lens for a given application. The second example below is an extension of the procedure, which will show how to circularize an elliptical beam.
Example 1: Collimating a Diverging Beam
- Laser Diode to be Used: L780P010
- Desired Collimated Beam Diameter: Ø3 mm (Major Axis)
When choosing a collimation lens, it is essential to know the divergence angle of the source being used and the desired output diameter. The specifications for the L780P010 laser diode indicate that the typical parallel and perpendicular FWHM beam divergences are 8° and 30°, respectively. Therefore, as the light diverges, an elliptical beam will result. To collect as much light as possible during the collimation process, consider the larger of these two divergence angles in any calculations (i.e., in this case, use 30°). If you wish to convert your elliptical beam into a round one, we suggest using an anamorphic prism pair, which magnifies one axis of your beam; for details, see Example 2 below.
Assuming that the thickness of the lens is small compared to the radius of curvature, the thin lens approximation can be used to determine the appropriate focal length for the asphere. Assuming a divergence angle of 30° (FWHM) and desired beam diameter of 3 mm:
 |
 |
||
| Θ = Divergence Angle | Ø = Beam Diameter | f = Focal Length | r = Collimated Beam Radius = Ø/2 |
Note that the focal length is generally not equal to the needed distance between the light source and the lens.
With this information known, it is now time to choose the appropriate collimating lens. Thorlabs offers a large selection of aspheric lenses. For this application, the ideal lens is a molded glass aspheric lens with focal length near 5.6 mm and our -B antireflection coating, which covers 780 nm. The C171TMD-B (mounted) or 354171-B (unmounted) aspheric lenses have a focal length of 6.20 mm, which will result in a collimated beam diameter (major axis) of 3.3 mm. Next, check to see if the numerical aperture (NA) of the diode is smaller than the NA of the lens:
0.30 = NALens > NADiode ≈ sin(15°) = 0.26
Up to this point, we have been using the full-width at half maximum (FWHM) beam diameter to characterize the beam. However, a better practice is to use the 1/e2 beam diameter. For a Gaussian beam profile, the 1/e2 diameter is almost equal to 1.7X the FWHM diameter. The 1/e2 beam diameter therefore captures more of the laser diode's output light (for greater power delivery) and minimizes far-field diffraction (by clipping less of the incident light).
A good rule of thumb is to pick a lens with an NA twice that of the laser diode NA. For example, either the A390-B or the A390TM-B could be used as these lenses each have an NA of 0.53, which is more than twice the approximate NA of our laser diode (0.26). These lenses each have a focal length of 4.6 mm, resulting in an approximate major beam diameter of 2.5 mm. In general, using a collimating lens with a short focal length will result in a small collimated beam diameter and a large beam divergence, while a lens with a large focal length will result in a large collimated beam diameter and a small divergence.
Example 2: Circularizing an Elliptical Beam
Using the laser diode and aspheric lens chosen above, we can use an anamorphic prism pair to convert our collimated, elliptical beam into a circular beam.
Whereas earlier we considered only the larger divergence angle, we now look at the smaller beam divergence of 8°. From this, and using the effective focal length of the A390-B aspheric lens chosen in Example 1, we can determine the length of the semi-minor axis of the elliptical beam after collimation:
r' = f * tan(Θ'/2) = 4.6 mm * tan(4°) = 0.32 mm
The minor beam diameter is double the semi-minor axis, or 0.64 mm. In order to magnify the minor diameter to be equal to the major diameter of 2.5 mm, we will need an anamorphic prism pair that yields a magnification of 3.9. Thorlabs offers both mounted and unmounted prism pairs. Mounted prism pairs provide the benefit of a stable housing to preserve alignment, while unmounted prism pairs can be positioned at any angle to achieve the exact desired magnification.
The PS883-B mounted prism pair provides a magnification of 4.0 for a 950 nm wavelength beam. Because shorter wavelengths undergo greater magnification when passing through the prism pair, we can expect our 780 nm beam to be magnified by slightly more than 4.0X. Thus, the beam will still maintain a small degree of ellipticity.
Alternatively, we can use the PS871-B unmounted prism pair to achieve the precise magnification of the minor diameter necessary to produce a circular beam. Using the data available here, we see that the PS871-B achieves a magnification of 4.0 when the prisms are positioned at the following angles for a 670 nm wavelength beam:
| α1: +34.608° | α2: -1.2455° |
Refer to the diagram to the right for α1 and α2 definitions. Our 780 nm laser will experience slightly less magnification than a 670 nm beam passing through the prisms at these angles. Some trial and error may be required to achieve the exact desired magnification. In general:
- To increase magnification, rotate the first prism clockwise (increasing α1) and rotate the second prism counterclockwise (decreasing α2).
- To reduce magnification, rotate the first prism counterclockwise (decreasing α1) and rotate the second prism clockwise (increasing α2).
| Posted Comments: | |
user
(posted 2023-10-11 12:47:32.913) Are the Graphs for the reflectance at 60° AOI measured for unpolarized light? If yes, does it mean that I get a reflectance of around 6% for S-polarization? jpolaris
(posted 2023-10-20 04:21:39.0) Thank you for contacting Thorlabs. Yes, the reflectance plots on the Graphs tab all correspond to unpolarized light. The polarization-dependent reflectance will vary somewhat over the 650 nm - 1050 nm range, but it is safe to assume it will be < 6%, with an average ~3% for S-polarized light. Veit Blümel
(posted 2023-01-13 12:20:14.107) Sehr geehrte Damen und Herren,
in der Packung war nur ein Prisma, obwohl ein Prismenpaar angegeben ist. Firma JENOPTIK Optical Systems GmbH, Lieferschein Nr. MP22459841. Könnten Sie das 2. Prisma bitte nachsenden?
Vielen Dank für Ihre Bemühungen
MfG Veit Blümel cdolbashian
(posted 2023-01-18 04:47:30.0) Thank you for contacting Thorlabs with this request! We will get you in contact with your local Thorlabs office to replace your missing prism. David Lowndes
(posted 2021-10-26 08:52:38.47) Could you please send me the raw data from the plots shown on the "Beam Expansion" tab, showing the alpha1 and alpha2 angles required for a given magnification. YLohia
(posted 2021-11-24 10:41:53.0) Thank you for contacting Thorlabs. We have updated the web documentation to include a download link to the raw data for those plots. Tanakrit Mamee
(posted 2020-12-08 01:18:38.443) Could you please provide the cw damage threshold of the product? YLohia
(posted 2020-12-08 02:30:33.0) Hello, thank you for contacting Thorlabs. I have reached out to you directly to discuss your application. Justin Molloy
(posted 2019-08-28 05:12:30.04) To produce an (approximately) circularized beam from a laser diode it would be helpful to have a threaded coupling on the input side (as well at the output side) of this mounted anamorphic prism pair (PS875-A). Then it would be easier to couple it to the laser-diode cage mount (LDH56-P2) using the cage plate translator (CPX1) to offset the input window so that it can be aligned (on-axis) with the incoming (elliptical) laser beam. The current design seems to require a bit more hardware, leading to a less compact, and slightly less rigid, optical setup. YLohia
(posted 2019-08-28 03:13:38.0) Hello Justin, thank you for your feedback. The PS875-A can be coupled to the CPX1 by using the SM05A3 (the adapter would go into the internally SM05-threaded port of the PS875-A). Wenzel Jakob
(posted 2019-08-16 05:53:04.35) Dear Thorlabs support, I'm wondering if there is a recommended way to mount an anamorphic prism pair like PS879-A in a 30mm or 60mm cage system where the input beam is centered? This means that I would need a cage system element that can hold such a long 1 inch diameter element with a vertical offset of 5.85 mm relative to the optical axis. Any suggestions would be greatly appreciated! YLohia
(posted 2019-08-16 09:49:39.0) Hello, thank you for contacting Thorlabs. You can use the CPX1 or the LCPX1 translating cage segment plates to achieve over +/- 6mm offset. You would have to use this with an appropriate SM1 lens tube such as the SM1L15. Michael Levin
(posted 2019-06-13 10:48:31.887) Hello Thorlabs team,
what is the distance between the middle of the entrance slit and the axis of the case in which the prisms are mounted? Is this distance the same for all prism pairs? To avoid confusion: I'm talking about mechanical dimensions only. For some reason this dimension is missing from mechanical drawings.
Best regards,
Michael YLohia
(posted 2019-06-13 12:06:57.0) Hello Michael, thank you for contacting Thorlabs. This distance is 5.08mm for the Mag 3.5 prism pairs. This number changes as the magnification changes and can be found using the Solidworks file that we provide. asckinha
(posted 2018-11-06 11:20:36.39) Hello,
Is it possible with the PS872-C to obtain a magnification of less than 2 reducing alpha1 and alpha2 even further?
We're aiming a magnification of 1.4 approximately.
Best Regards, nbayconich
(posted 2018-11-07 01:19:49.0) Thank you for contacting Thorlabs. Yes the magnification can be adjusted to less than 2X or even less than 1.4X by adjusting either angles (alpha1 or alpha2). I will reach out to you directly with more information. ralley
(posted 2018-03-27 14:46:38.25) I was curious if you have a technical guide or note for building Anamorphic beam expander? I need to build custom optics for fixing elliptical beams. With fine details on theory. Best regards. YLohia
(posted 2018-03-30 04:29:35.0) Hello, thank you for leaving your feedback. Currently, we do not have a specific guide for building two-dimensional beam expanders with these since the typical application for anamorphic prism pairs is for beam shaping, which is beam expansion in one dimension. If that is what you mean, then we do have a guide here: https://www.thorlabs.com/images/TabImages/Circularization_of_Elliptical_Beams.pdf. We will reach out to you directly to discuss the possibility of offering custom optics. w.husson
(posted 2018-01-24 16:05:28.673) Hello,
I need to use your prism pair backwards to reduce the beam size on the output of a laser diode (520nm).
Unfortunately it forces us to work on S polarization, and according to our measurements, the AR coating is totally inefficient with a very bad throughput.
Do you have a solution?
Best regards. tfrisch
(posted 2018-01-26 02:30:18.0) Hello, thank you for contacting Thorlabs. I would recommend using WPH05M-514 to rotate the polarization state 90 degrees which will not change the geometric shape of the beam. Then you will be working with the P state on the prism pair. I will reach out to you directly to discuss your application. rsubkh
(posted 2016-10-05 09:23:25.267) Dear Sirs,
I would like to know what is the optical transmission of the pair of anamorphic prisms at 355nm with AR coating applied.
Kind regards,
Ruslan jlow
(posted 2016-10-05 02:20:24.0) Response from Jeremy at Thorlabs: The transmission is estimated to be around 70% at 355nm. volker
(posted 2016-06-17 16:03:09.493) would you be able to supply the prisms with no coating on the surface with Brewster angle incidence? We are planning to use them intra-cavity in a laser system, where we found that the losses of the AR coating at the Brewster side are too high >3%
One the side with 0degree AOI we still require the AR coating. besembeson
(posted 2016-06-21 02:40:44.0) Response from Bweh at Thorlabs USA: Yes this is possible. I will contact you. quig5862
(posted 2016-05-30 23:10:02.46) Hi i dont know much about optics. lenses, etc. But would anything u sell be of use for a Home projector. Basically I'm looking for a cheap option to view 21:9 content with a 16:9 projector and read that you can use prism lens for horizontal stretching. besembeson
(posted 2016-06-02 01:12:34.0) Response from Bweh at Thorlabs USA: In principle you can, as these are designed to stretch a beam or image rays in one direction. But the ones we carry are mostly designed for laboratory use to stretch the elliptical output from a diode laser in one direction. You will have to construct an imaging system to achieve that. gotodani
(posted 2016-02-01 03:04:16.873) Hello
We are currently using the Anamorphic Prism Pairs (PS879-B) for beam shaping of two lasers (766 and 780 nm). Both lasers are combined before entering the prism pairs. Our question is will these two lasers (which are combined into one single beam) be separated into two beams after passing through the prism pairs because of the diffraction? besembeson
(posted 2016-02-04 11:41:23.0) Response from Bweh at Thorlabs USA: Yes this will happen. The magnification is also wavelength dependent so the beams will be stretched differently through the prism pair. If you can refocus the beam before the shaping optics, a cylindrical lens combination (achromatic) will be more appropriate. mmodena
(posted 2014-02-04 12:16:46.72) Hi,
I need to shape a circular beam into an elliptical one. As I read, I can use the prism pair in reverse configuration to achieve this. My only concern regards the ar coating: should I purchase an uncoated prism pair?
Thank you,
Mario Modena besembeson
(posted 2014-02-07 04:45:32.0) Response from Bweh E at Thorlabs: I think it will be better to use the coated ones, since two of the four surfaces will coincide with the optimal AR coating angle. The other two will be off but based on the reflectivity specifications (see "Reflectivity tab at the following link: http://www.thorlabs.de/newgrouppage9.cfm?objectgroup_id=149&pn=PS873-A) you should still be okay. christian
(posted 2013-12-05 10:01:24.507) I am using using the F810SMA-635 Collimator(with LPM-635-SMA Diode)with a beam aprox. 17mm in diameter. Are there larger prism pairs or other possibilities for transforming to a cylindrical beam? cdaly
(posted 2013-12-05 02:35:27.0) Response from Chris at Thorlabs: Thank you for your inquiry. I would recommend using a par of cylindrical lenses to manipulate the beam this way. You can use two which are position to share a focal point. If they are lined up the same way, it will expand or reduce the beam in one axis by the ratio of the focal lengths of the lenses. bdada
(posted 2012-03-15 15:06:00.0) Response from Buki at Thorlabs to sechaniz: As an update, the damage threshold of the uncoated prism is 10 J/cm2, 10 nsec, 10 Hz @ 1064nm. bdada
(posted 2012-03-15 12:50:00.0) Response from Buki at Thorlabs to sechaniz:
Thank you for your feedback. We don't have damage threshold test data for the anamorphic prism pairs, but the damage threshold limit for the coated prisms is determined by the AR coating, which is nominally 100 mJ/cm^2 for a 10 ns pulse or 100W/cm^2 at 1064nm.
Based on the information you provided, your beam should have a density of about 12W/cm^2, which is below the damage threshold of the coating.
Please contact TechSupport@thorlabs.com if you have any questions. sechaniz
(posted 2012-03-12 15:41:47.0) Could you please let me know what is the damage threshold of these prisms? In particular, I'd like to use them with an 808 nm laser diode with 1.2 W of power and a beam of 5 x 2 mm. Would this be possible?
Thank you. bdada
(posted 2012-01-27 02:18:00.0) Response from Buki at Thorlabs:
Thank you for using our feedback forum. Anamorphic prism pairs can be used in reverse to convert a circular beam into an elliptical one. We do not anticipate any issues. Please contact TechSupport@thorlabs.com if you have additional questions or want to discuss your application further. franxm
(posted 2012-01-13 14:34:45.0) Any issues if the input and output are reversed (i.e., using the anamorphic prism pair to convert a circular beam into an elliptical one)? bdada
(posted 2011-03-08 18:09:00.0) Response from Buki:
Thank you for your request. We do manufacture custom prisms and we will contact you directly to discuss your application. spotnis
(posted 2011-03-07 10:26:22.0) Does thorlab manufacture custom prisms with a wide exit aperture? Im looking for a prism pair which has 10-15x expansion and a 20mm exit aperture. Thorlabs
(posted 2010-10-29 15:37:16.0) Response from Javier at Thorlabs to kjsong: Thank you very much for your feedback. I will discuss adding -A versions of our anamorphic prism pairs with our optics department. I will keep you updated. kjsong
(posted 2010-10-29 13:41:46.0) I am working with 635nm light. I would like to have PS875-A. But its not listed. It seems odd that there are laser diodes at 400nm and 633nm but no AR coating to cover that range. Its 4 surfaces in an anamorphic prism! That reflects a lot of light! Tyler
(posted 2008-09-09 17:02:54.0) A response from Tyler at Thorlabs to rieko.verhagen: We dont have damage threshold test data for the anamorphic prism pairs at 1600 nm. However, the damage threshold limit is determined by the AR coating (if present), which is nominally 100 mJ/cm^2 for 10 ns pulse. Does your application require a higher damage threshold? rieko.verhagen
(posted 2008-09-05 07:50:41.0) Thorlabs,
Could you please provide me with the damage threshold for nanosecond pulsed laser operation around 1600nm for the PS872-C anamorphic prism pair?
With kind regards,
Rieko Verhagen |
Selection Guide for Prisms
Thorlabs offers a wide variety of prisms, which can be used to reflect, invert, rotate, disperse, steer, and collimate light. For prisms and substrates not listed below, please contact Tech Support.
Beam Steering Prisms
| Prism | Material | Deviation | Invert | Reverse or Rotate | Illustration | Applications |
|---|---|---|---|---|---|---|
| Right Angle Prisms | N-BK7, UV Fused Silica, Calcium Fluoride, or Zinc Selenide | 90° | 90° | No |  |
90° reflector used in optical systems such as telescopes and periscopes. |
| 180° | 180° | No |  |
180° reflector, independent of entrance beam angle. Acts as a non-reversing mirror and can be used in binocular configurations. |
||
| TIR Retroreflectors (Unmounted and Mounted) and Specular Retroreflectors (Unmounted and Mounted) |
N-BK7 | 180° | 180° | No |  |
180° reflector, independent of entrance beam angle. Beam alignment and beam delivery. Substitute for mirror in applications where orientation is difficult to control. |
| Unmounted Penta Prisms and Mounted Penta Prisms |
N-BK7 | 90° | No | No | 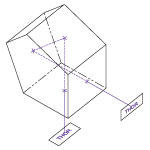 |
90° reflector, without inversion or reversal of the beam profile. Can be used for alignment and optical tooling. |
| Roof Prisms | N-BK7 | 90° | 90° | 180o Rotation | 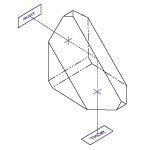 |
90° reflector, inverted and rotated (deflected left to right and top to bottom). Can be used for alignment and optical tooling. |
| Unmounted Dove Prisms and Mounted Dove Prisms |
N-BK7 | No | 180° | 2x Prism Rotation |  |
Dove prisms may invert, reverse, or rotate an image based on which face the light is incident on. Prism in a beam rotator orientation. |
| 180° | 180° | No |  |
Prism acts as a non-reversing mirror. Same properties as a retroreflector or right angle (180° orientation) prism in an optical setup. |
||
| Wedge Prisms | N-BK7 | Models Available from 2° to 10° | No | No |  |
Beam steering applications. By rotating one wedged prism, light can be steered to trace the circle defined by 2 times the specified deviation angle. |
| No | No |  |
Variable beam steering applications. When both wedges are rotated, the beam can be moved anywhere within the circle defined by 4 times the specified deviation angle. |
|||
| Coupling Prisms | Rutile (TiO2) or GGG | Variablea | No | No | 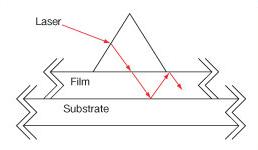 |
High index of refraction substrate used to couple light into films. Rutile used for nfilm > 1.8 GGG used for nfilm < 1.8 |
Dispersive Prisms
| Prism | Material | Deviation | Invert | Reverse or Rotate | Illustration | Applications |
|---|---|---|---|---|---|---|
| Equilateral Prisms | F2, N-F2, N-SF11, Calcium Fluoride, or Zinc Selenide |
Variablea | No | No |  |
Dispersion prisms are a substitute for diffraction gratings. Use to separate white light into visible spectrum. |
| Dispersion Compensating Prism Pairs | Fused Silica, Calcium Fluoride, SF10, or N-SF14 | Variable Vertical Offset | No | No | 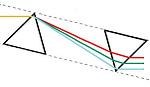 |
Compensate for pulse broadening effects in ultrafast laser systems. Can be used as an optical filter, for wavelength tuning, or dispersion compensation.
|
| Pellin Broca Prisms | N-BK7, UV Fused Silica, or Calcium Fluoride |
90° | 90° | No | 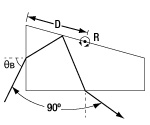 |
Ideal for wavelength separation of a beam of light, output at 90°. Used to separate harmonics of a laser or compensate for group velocity dispersion. |
Beam Manipulating Prisms
| Prism | Material | Deviation | Invert | Reverse or Rotate | Illustration | Applications |
|---|---|---|---|---|---|---|
| Anamorphic Prism Pairs | N-KZFS8 or N-SF11 |
Variable Vertical Offset | No | No |  |
Variable magnification along one axis. Collimating elliptical beams (e.g., laser diodes) Converts an elliptical beam into a circular beam by magnifying or contracting the input beam in one axis. |
| Axicons (UVFS, ZnSe) | UV Fused Silica or Zinc Selenide |
Variablea | No | No |
Creates a conical, non-diverging beam with a Bessel intensity profile from a collimated source. |
Polarization Altering Prisms
| Prism | Material | Deviation | Invert | Reverse or Rotate | Illustration | Applications |
|---|---|---|---|---|---|---|
| Glan-Taylor, Glan-Laser, and α-BBO Glan-Laser Polarizers | Glan-Taylor: Calcite Glan-Laser: α-BBO or Calcite |
p-pol. - 0° s-pol. - 112°a |
No | No |  |
Double prism configuration and birefringent calcite produce extremely pure linearly polarized light. Total Internal Reflection of s-pol. at the gap between the prism while p-pol. is transmitted. |
| Rutile Polarizers | Rutile (TiO2) | s-pol. - 0° p-pol. absorbed by housing |
No | No |  |
Double prism configuration and birefringent rutile (TiO2) produce extremely pure linearly polarized light. Total Internal Reflection of p-pol. at the gap between the prisms while s-pol. is transmitted.
|
| Double Glan-Taylor Polarizers | Calcite | p-pol. - 0° s-pol. absorbed by housing |
No | No |  |
Triple prism configuration and birefringent calcite produce maximum polarized field over a large half angle. Total Internal Reflection of s-pol. at the gap between the prism while p-pol. is transmitted. |
| Glan Thompson Polarizers | Calcite | p-pol. - 0° s-pol. absorbed by housing |
No | No |  |
Double prism configuration and birefringent calcite produce a polarizer with the widest field of view while maintaining a high extinction ratio. Total Internal Reflection of s-pol. at the gap between the prism while p-pol. is transmitted. |
| Wollaston Prisms and Wollaston Polarizers |
Quartz, Magnesium Fluoride, α-BBO, Calcite, Yttrium Orthovanadate | Symmetric p-pol. and s-pol. deviation angle |
No | No |  |
Double prism configuration and birefringent calcite produce the widest deviation angle of beam displacing polarizers. s-pol. and p-pol. deviate symmetrically from the prism. Wollaston prisms are used in spectrometers and polarization analyzers. |
| Rochon Prisms | Magnesium Fluoride or Yttrium Orthovanadate |
Ordinary Ray: 0° Extraordinary Ray: deviation angle |
No | No |  |
Double prism configuration and birefringent MgF2 or YVO4 produce a small deviation angle with a high extinction ratio. Extraordinary ray deviates from the input beam's optical axis, while ordinary ray does not deviate. |
| Beam Displacing Prisms | Calcite | 2.7 or 4.0 mm Beam Displacement | No | No |  |
Single prism configuration and birefringent calcite separate an input beam into two orthogonally polarized output beams. s-pol. and p-pol. are displaced by 2.7 or 4.0 mm. Beam displacing prisms can be used as polarizing beamsplitters where 90o separation is not possible. |
| Fresnel Rhomb Retarders | N-BK7 | Linear to circular polarization Vertical Offset |
No | No |  |
λ/4 Fresnel Rhomb Retarder turns a linear input into circularly polarized output. Uniform λ/4 retardance over a wider wavelength range compared to birefringent wave plates. |
| Rotates linearly polarized light 90° | No | No |  |
λ/2 Fresnel Rhomb Retarder rotates linearly polarized light 90°. Uniform λ/2 retardance over a wider wavelength range compared to birefringent wave plates. |
Beamsplitter Prisms
| Prism | Material | Deviation | Invert | Reverse or Rotate | Illustration | Applications |
|---|---|---|---|---|---|---|
| Beamsplitter Cubes | N-BK7 | 50:50 splitting ratio, 0° and 90° s- and p- pol. within 10% of each other |
No | No |  |
Double prism configuration and dielectric coating provide 50:50 beamsplitting nearly independent of polarization. Non-polarizing beamsplitter over the specified wavelength range. |
| Polarizing Beamsplitter Cubes | N-BK7, UV Fused Silica, or N-SF1 | p-pol. - 0° s-pol. - 90° |
No | No |  |
Double prism configuration and dielectric coating transmit p-pol. light and reflect s-pol. light. For highest polarization use the transmitted beam. |

These unmounted prism pairs are available uncoated or with an antireflection coating for the 350 - 700 nm (-A), 650 - 1050 nm (-B) or 1050 - 1700 nm (-C) range. The anamorphic expansion (one-dimensional expansion) can be adjusted by changing the angles and the offset between the prisms. Please see the Beam Expansion tab for details.

| Item # | Anamorphic Magnificationa |
Input Offset, Lb (mm) |
|---|---|---|
| PS875-A | 2.0 | 4.7 |
| PS879-A | 3.0 | 5.85 |
| PS883-A | 4.0 | 5.81 |

| Item # | Anamorphic Magnificationa |
Input Offset, Lb (mm) |
|---|---|---|
| PS875-B | 2.0 | 3.7 |
| PS877-B | 2.5 | 5.15 |
| PS879-B | 3.0 | 5.43 |
| PS880-B | 3.2 | 5.63 |
| PS881-B | 3.5 | 6.0 |
| PS883-B | 4.0 | 6.06 |
 Products Home
Products Home















 Click to Enlarge
Click to Enlarge













 Zoom
Zoom


 Anamorphic Prism Pairs
Anamorphic Prism Pairs