Mounted Superachromatic Wave Plates
- Quarter- or Half-Wave Plates
- Extremely Flat Retardance Over a Super Broadband Range
- Ø1" Engraved Housing with Ø10.0 mm Clear Aperture
SAQWP05M-700
Quarter-Wave Plate for 325 - 1100 nm
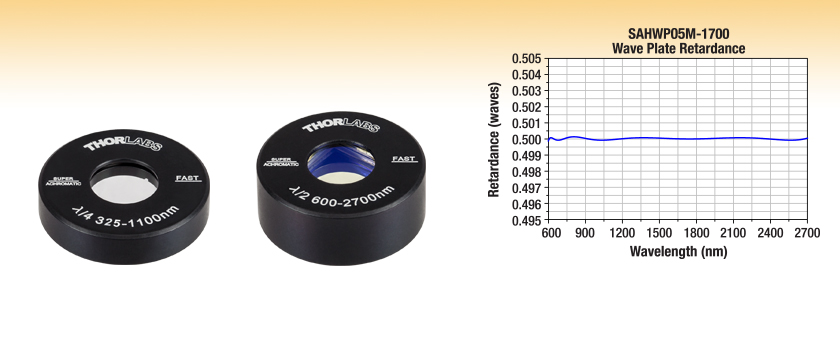
SAHWP05M-1700
Half-Wave Plate for 600 - 2700 nm

Please Wait
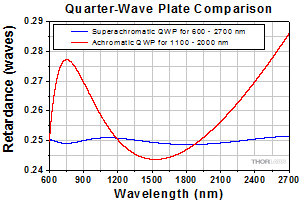
These plots compare the theoretical performance of two of our superachromatic wave plates to their closest equivalent achromatic wave plates. On the left, the SAQWP05M-1700 and AQWP05M-1600 quarter-wave plates are shown, and on the right, our SAHWP05M-1700 and AHWP05M-1600 half-wave plates are shown. The superachromatic wave plates provide superior retardance flatness and accuracy over a much broader range. For details on the retardance curves for our superachromatic wave plates, please see the info icons (![]() ) below.
) below.
| Webpage Features | |
|---|---|
| Clicking this icon opens a window that contains specifications and mechanical drawings. | |
| Clicking this icon allows you to download our standard support documentation. | |
Features
- Accurate Retardance Over a Super Broadband Range
- Quarter-Wave Plates for 325 - 1100 nm or 600 - 2700 nm
- Half-Wave Plates for 310 - 1100 nm or 600 - 2700 nm
- Ø10.0 mm Clear Aperture
- Mounted in a Ø1" Engraved Housing
- Six-Element, Optically Cemented Design
- Custom Options Available, Please Contact Tech Support for More Information
Thorlabs' Superachromatic Wave Plates offer extremely flat quarter-wave or half-wave retardance over an extremely broadband range. Quarter-wave plates are available for 325 - 1100 nm or 600 - 2700 nm and half-wave plates are available for 310 - 1100 nm or 600 - 2700 nm. Based on the design by Pancharatnama, each consists of three quartz and three magnesium fluoride (MgF2) plates that are optically cemented to maximize transmission and carefully aligned to minimize the wavelength dependence of the retardance. Compared to other wave plates, superachromatic wave plates offer significantly flatter retardance over a significantly broader range. The plots to the right compare them to achromatic wave plates, the closest equivalent optics.
Our superachromatic wave plates are designed for use at normal incidence and feature a clear aperture of Ø10.0 mm. As shown in the image at the top of the page, they are packaged in Ø1" anodized aluminum housings engraved with the retardance value, the wavelength range, and the fast axis orientation at 633 nm (see below for information regarding the fast axis orientation at other wavelengths). Since the housings are unthreaded, we recommend securing them into our SM1-threaded rotation mounts with an SM1RR Retaining Ring.
The 1/2" x 1/2" six-element optical stack is secured into the housing by a retaining ring. We do not recommend disturbing this retaining ring, as it is likely to affect the optical alignment of the fast axis of the wave plate. The orientation of the fast axis must be determined by the user if the wave plate is removed from the mount, as there is no physical reference on the optic.
Thorlabs can also produce custom wave plates with no AR coating, a different AR coating, or a different design wavelength than those offered below. Please contact Tech Support with inquiries.
Wavelength Dependence of Fast Axis
Each housing is engraved with the orientation of the fast axis as determined at 633 nm. As a consequence of the six-element design that is required for obtaining super broadband operation, the fast axis orientation slightly varies with wavelength. The absolute magnitude of this variation over the entire operating wavelength range is less than 1° for the 600 - 2700 nm wave plates and less than 5° for the 310 - 1100 nm and 325 - 1100 nm wave plates. Please see the info icons (![]() ) below for wavelength-dependent plots of the fast axis orientation. Our other wave plates do not exhibit any wavelength dependence of the fast axis orientation.
) below for wavelength-dependent plots of the fast axis orientation. Our other wave plates do not exhibit any wavelength dependence of the fast axis orientation.
a. Pancharatnam, S., Proceedings of the Indian Academy of Sciences 41, 130 (1955).
| Wave Plate Selection Guide | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Achromatic | Superachromatic | Quartz Zero-Order Half-Wave |
Quartz Zero-Order Quarter-Wave |
Polymer Zero-Order Half-Wave |
Polymer Zero-Order Quarter-Wave |
Low-Order | Multi-Order | Dual Wavelength | Telecom | Polarization Optics |
| Damage Threshold Specification | |
|---|---|
| Pulsed | 0.5 J/cm² (1064 nm, 10 ns, 10 Hz, Ø1 mm) |
| CWa | 1500 W/cm (1064 nm, Ø0.091 mm) |
Damage Threshold Data for Thorlabs' Mounted Superachromatic Wave Plates
The specification to the right is measured data for Thorlabs' mounted superachromatic wave plates. The damage threshold specification is the same for all of our superachromatic plates.
Laser Induced Damage Threshold Tutorial
The following is a general overview of how laser induced damage thresholds are measured and how the values may be utilized in determining the appropriateness of an optic for a given application. When choosing optics, it is important to understand the Laser Induced Damage Threshold (LIDT) of the optics being used. The LIDT for an optic greatly depends on the type of laser you are using. Continuous wave (CW) lasers typically cause damage from thermal effects (absorption either in the coating or in the substrate). Pulsed lasers, on the other hand, often strip electrons from the lattice structure of an optic before causing thermal damage. Note that the guideline presented here assumes room temperature operation and optics in new condition (i.e., within scratch-dig spec, surface free of contamination, etc.). Because dust or other particles on the surface of an optic can cause damage at lower thresholds, we recommend keeping surfaces clean and free of debris. For more information on cleaning optics, please see our Optics Cleaning tutorial.
Testing Method
Thorlabs' LIDT testing is done in compliance with ISO/DIS 11254 and ISO 21254 specifications.
First, a low-power/energy beam is directed to the optic under test. The optic is exposed in 10 locations to this laser beam for 30 seconds (CW) or for a number of pulses (pulse repetition frequency specified). After exposure, the optic is examined by a microscope (~100X magnification) for any visible damage. The number of locations that are damaged at a particular power/energy level is recorded. Next, the power/energy is either increased or decreased and the optic is exposed at 10 new locations. This process is repeated until damage is observed. The damage threshold is then assigned to be the highest power/energy that the optic can withstand without causing damage. A histogram such as that below represents the testing of one BB1-E02 mirror.

The photograph above is a protected aluminum-coated mirror after LIDT testing. In this particular test, it handled 0.43 J/cm2 (1064 nm, 10 ns pulse, 10 Hz, Ø1.000 mm) before damage.
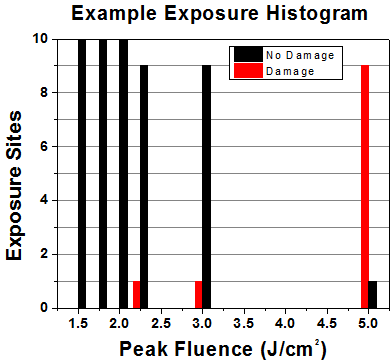
| Example Test Data | |||
|---|---|---|---|
| Fluence | # of Tested Locations | Locations with Damage | Locations Without Damage |
| 1.50 J/cm2 | 10 | 0 | 10 |
| 1.75 J/cm2 | 10 | 0 | 10 |
| 2.00 J/cm2 | 10 | 0 | 10 |
| 2.25 J/cm2 | 10 | 1 | 9 |
| 3.00 J/cm2 | 10 | 1 | 9 |
| 5.00 J/cm2 | 10 | 9 | 1 |
According to the test, the damage threshold of the mirror was 2.00 J/cm2 (532 nm, 10 ns pulse, 10 Hz, Ø0.803 mm). Please keep in mind that these tests are performed on clean optics, as dirt and contamination can significantly lower the damage threshold of a component. While the test results are only representative of one coating run, Thorlabs specifies damage threshold values that account for coating variances.
Continuous Wave and Long-Pulse Lasers
When an optic is damaged by a continuous wave (CW) laser, it is usually due to the melting of the surface as a result of absorbing the laser's energy or damage to the optical coating (antireflection) [1]. Pulsed lasers with pulse lengths longer than 1 µs can be treated as CW lasers for LIDT discussions.
When pulse lengths are between 1 ns and 1 µs, laser-induced damage can occur either because of absorption or a dielectric breakdown (therefore, a user must check both CW and pulsed LIDT). Absorption is either due to an intrinsic property of the optic or due to surface irregularities; thus LIDT values are only valid for optics meeting or exceeding the surface quality specifications given by a manufacturer. While many optics can handle high power CW lasers, cemented (e.g., achromatic doublets) or highly absorptive (e.g., ND filters) optics tend to have lower CW damage thresholds. These lower thresholds are due to absorption or scattering in the cement or metal coating.

LIDT in linear power density vs. pulse length and spot size. For long pulses to CW, linear power density becomes a constant with spot size. This graph was obtained from [1].
Pulsed lasers with high pulse repetition frequencies (PRF) may behave similarly to CW beams. Unfortunately, this is highly dependent on factors such as absorption and thermal diffusivity, so there is no reliable method for determining when a high PRF laser will damage an optic due to thermal effects. For beams with a high PRF both the average and peak powers must be compared to the equivalent CW power. Additionally, for highly transparent materials, there is little to no drop in the LIDT with increasing PRF.
In order to use the specified CW damage threshold of an optic, it is necessary to know the following:
- Wavelength of your laser
- Beam diameter of your beam (1/e2)
- Approximate intensity profile of your beam (e.g., Gaussian)
- Linear power density of your beam (total power divided by 1/e2 beam diameter)
Thorlabs expresses LIDT for CW lasers as a linear power density measured in W/cm. In this regime, the LIDT given as a linear power density can be applied to any beam diameter; one does not need to compute an adjusted LIDT to adjust for changes in spot size, as demonstrated by the graph to the right. Average linear power density can be calculated using the equation below.

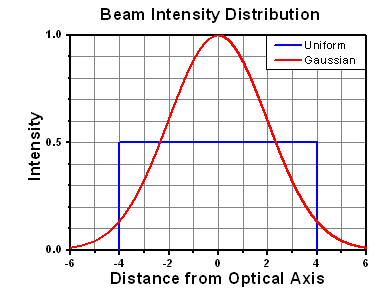
The calculation above assumes a uniform beam intensity profile. You must now consider hotspots in the beam or other non-uniform intensity profiles and roughly calculate a maximum power density. For reference, a Gaussian beam typically has a maximum power density that is twice that of the uniform beam (see lower right).
Now compare the maximum power density to that which is specified as the LIDT for the optic. If the optic was tested at a wavelength other than your operating wavelength, the damage threshold must be scaled appropriately. A good rule of thumb is that the damage threshold has a linear relationship with wavelength such that as you move to shorter wavelengths, the damage threshold decreases (i.e., a LIDT of 10 W/cm at 1310 nm scales to 5 W/cm at 655 nm):

While this rule of thumb provides a general trend, it is not a quantitative analysis of LIDT vs wavelength. In CW applications, for instance, damage scales more strongly with absorption in the coating and substrate, which does not necessarily scale well with wavelength. While the above procedure provides a good rule of thumb for LIDT values, please contact Tech Support if your wavelength is different from the specified LIDT wavelength. If your power density is less than the adjusted LIDT of the optic, then the optic should work for your application.
Please note that we have a buffer built in between the specified damage thresholds online and the tests which we have done, which accommodates variation between batches. Upon request, we can provide individual test information and a testing certificate. The damage analysis will be carried out on a similar optic (customer's optic will not be damaged). Testing may result in additional costs or lead times. Contact Tech Support for more information.
Pulsed Lasers
As previously stated, pulsed lasers typically induce a different type of damage to the optic than CW lasers. Pulsed lasers often do not heat the optic enough to damage it; instead, pulsed lasers produce strong electric fields capable of inducing dielectric breakdown in the material. Unfortunately, it can be very difficult to compare the LIDT specification of an optic to your laser. There are multiple regimes in which a pulsed laser can damage an optic and this is based on the laser's pulse length. The highlighted columns in the table below outline the relevant pulse lengths for our specified LIDT values.
Pulses shorter than 10-9 s cannot be compared to our specified LIDT values with much reliability. In this ultra-short-pulse regime various mechanics, such as multiphoton-avalanche ionization, take over as the predominate damage mechanism [2]. In contrast, pulses between 10-7 s and 10-4 s may cause damage to an optic either because of dielectric breakdown or thermal effects. This means that both CW and pulsed damage thresholds must be compared to the laser beam to determine whether the optic is suitable for your application.
| Pulse Duration | t < 10-9 s | 10-9 < t < 10-7 s | 10-7 < t < 10-4 s | t > 10-4 s |
|---|---|---|---|---|
| Damage Mechanism | Avalanche Ionization | Dielectric Breakdown | Dielectric Breakdown or Thermal | Thermal |
| Relevant Damage Specification | No Comparison (See Above) | Pulsed | Pulsed and CW | CW |
When comparing an LIDT specified for a pulsed laser to your laser, it is essential to know the following:

LIDT in energy density vs. pulse length and spot size. For short pulses, energy density becomes a constant with spot size. This graph was obtained from [1].
- Wavelength of your laser
- Energy density of your beam (total energy divided by 1/e2 area)
- Pulse length of your laser
- Pulse repetition frequency (prf) of your laser
- Beam diameter of your laser (1/e2 )
- Approximate intensity profile of your beam (e.g., Gaussian)
The energy density of your beam should be calculated in terms of J/cm2. The graph to the right shows why expressing the LIDT as an energy density provides the best metric for short pulse sources. In this regime, the LIDT given as an energy density can be applied to any beam diameter; one does not need to compute an adjusted LIDT to adjust for changes in spot size. This calculation assumes a uniform beam intensity profile. You must now adjust this energy density to account for hotspots or other nonuniform intensity profiles and roughly calculate a maximum energy density. For reference a Gaussian beam typically has a maximum energy density that is twice that of the 1/e2 beam.
Now compare the maximum energy density to that which is specified as the LIDT for the optic. If the optic was tested at a wavelength other than your operating wavelength, the damage threshold must be scaled appropriately [3]. A good rule of thumb is that the damage threshold has an inverse square root relationship with wavelength such that as you move to shorter wavelengths, the damage threshold decreases (i.e., a LIDT of 1 J/cm2 at 1064 nm scales to 0.7 J/cm2 at 532 nm):

You now have a wavelength-adjusted energy density, which you will use in the following step.
Beam diameter is also important to know when comparing damage thresholds. While the LIDT, when expressed in units of J/cm², scales independently of spot size; large beam sizes are more likely to illuminate a larger number of defects which can lead to greater variances in the LIDT [4]. For data presented here, a <1 mm beam size was used to measure the LIDT. For beams sizes greater than 5 mm, the LIDT (J/cm2) will not scale independently of beam diameter due to the larger size beam exposing more defects.
The pulse length must now be compensated for. The longer the pulse duration, the more energy the optic can handle. For pulse widths between 1 - 100 ns, an approximation is as follows:

Use this formula to calculate the Adjusted LIDT for an optic based on your pulse length. If your maximum energy density is less than this adjusted LIDT maximum energy density, then the optic should be suitable for your application. Keep in mind that this calculation is only used for pulses between 10-9 s and 10-7 s. For pulses between 10-7 s and 10-4 s, the CW LIDT must also be checked before deeming the optic appropriate for your application.
Please note that we have a buffer built in between the specified damage thresholds online and the tests which we have done, which accommodates variation between batches. Upon request, we can provide individual test information and a testing certificate. Contact Tech Support for more information.
[1] R. M. Wood, Optics and Laser Tech. 29, 517 (1998).
[2] Roger M. Wood, Laser-Induced Damage of Optical Materials (Institute of Physics Publishing, Philadelphia, PA, 2003).
[3] C. W. Carr et al., Phys. Rev. Lett. 91, 127402 (2003).
[4] N. Bloembergen, Appl. Opt. 12, 661 (1973).
In order to illustrate the process of determining whether a given laser system will damage an optic, a number of example calculations of laser induced damage threshold are given below. For assistance with performing similar calculations, we provide a spreadsheet calculator that can be downloaded by clicking the button to the right. To use the calculator, enter the specified LIDT value of the optic under consideration and the relevant parameters of your laser system in the green boxes. The spreadsheet will then calculate a linear power density for CW and pulsed systems, as well as an energy density value for pulsed systems. These values are used to calculate adjusted, scaled LIDT values for the optics based on accepted scaling laws. This calculator assumes a Gaussian beam profile, so a correction factor must be introduced for other beam shapes (uniform, etc.). The LIDT scaling laws are determined from empirical relationships; their accuracy is not guaranteed. Remember that absorption by optics or coatings can significantly reduce LIDT in some spectral regions. These LIDT values are not valid for ultrashort pulses less than one nanosecond in duration.

A Gaussian beam profile has about twice the maximum intensity of a uniform beam profile.
CW Laser Example
Suppose that a CW laser system at 1319 nm produces a 0.5 W Gaussian beam that has a 1/e2 diameter of 10 mm. A naive calculation of the average linear power density of this beam would yield a value of 0.5 W/cm, given by the total power divided by the beam diameter:

However, the maximum power density of a Gaussian beam is about twice the maximum power density of a uniform beam, as shown in the graph to the right. Therefore, a more accurate determination of the maximum linear power density of the system is 1 W/cm.
An AC127-030-C achromatic doublet lens has a specified CW LIDT of 350 W/cm, as tested at 1550 nm. CW damage threshold values typically scale directly with the wavelength of the laser source, so this yields an adjusted LIDT value:

The adjusted LIDT value of 350 W/cm x (1319 nm / 1550 nm) = 298 W/cm is significantly higher than the calculated maximum linear power density of the laser system, so it would be safe to use this doublet lens for this application.
Pulsed Nanosecond Laser Example: Scaling for Different Pulse Durations
Suppose that a pulsed Nd:YAG laser system is frequency tripled to produce a 10 Hz output, consisting of 2 ns output pulses at 355 nm, each with 1 J of energy, in a Gaussian beam with a 1.9 cm beam diameter (1/e2). The average energy density of each pulse is found by dividing the pulse energy by the beam area:

As described above, the maximum energy density of a Gaussian beam is about twice the average energy density. So, the maximum energy density of this beam is ~0.7 J/cm2.
The energy density of the beam can be compared to the LIDT values of 1 J/cm2 and 3.5 J/cm2 for a BB1-E01 broadband dielectric mirror and an NB1-K08 Nd:YAG laser line mirror, respectively. Both of these LIDT values, while measured at 355 nm, were determined with a 10 ns pulsed laser at 10 Hz. Therefore, an adjustment must be applied for the shorter pulse duration of the system under consideration. As described on the previous tab, LIDT values in the nanosecond pulse regime scale with the square root of the laser pulse duration:

This adjustment factor results in LIDT values of 0.45 J/cm2 for the BB1-E01 broadband mirror and 1.6 J/cm2 for the Nd:YAG laser line mirror, which are to be compared with the 0.7 J/cm2 maximum energy density of the beam. While the broadband mirror would likely be damaged by the laser, the more specialized laser line mirror is appropriate for use with this system.
Pulsed Nanosecond Laser Example: Scaling for Different Wavelengths
Suppose that a pulsed laser system emits 10 ns pulses at 2.5 Hz, each with 100 mJ of energy at 1064 nm in a 16 mm diameter beam (1/e2) that must be attenuated with a neutral density filter. For a Gaussian output, these specifications result in a maximum energy density of 0.1 J/cm2. The damage threshold of an NDUV10A Ø25 mm, OD 1.0, reflective neutral density filter is 0.05 J/cm2 for 10 ns pulses at 355 nm, while the damage threshold of the similar NE10A absorptive filter is 10 J/cm2 for 10 ns pulses at 532 nm. As described on the previous tab, the LIDT value of an optic scales with the square root of the wavelength in the nanosecond pulse regime:

This scaling gives adjusted LIDT values of 0.08 J/cm2 for the reflective filter and 14 J/cm2 for the absorptive filter. In this case, the absorptive filter is the best choice in order to avoid optical damage.
Pulsed Microsecond Laser Example
Consider a laser system that produces 1 µs pulses, each containing 150 µJ of energy at a repetition rate of 50 kHz, resulting in a relatively high duty cycle of 5%. This system falls somewhere between the regimes of CW and pulsed laser induced damage, and could potentially damage an optic by mechanisms associated with either regime. As a result, both CW and pulsed LIDT values must be compared to the properties of the laser system to ensure safe operation.
If this relatively long-pulse laser emits a Gaussian 12.7 mm diameter beam (1/e2) at 980 nm, then the resulting output has a linear power density of 5.9 W/cm and an energy density of 1.2 x 10-4 J/cm2 per pulse. This can be compared to the LIDT values for a WPQ10E-980 polymer zero-order quarter-wave plate, which are 5 W/cm for CW radiation at 810 nm and 5 J/cm2 for a 10 ns pulse at 810 nm. As before, the CW LIDT of the optic scales linearly with the laser wavelength, resulting in an adjusted CW value of 6 W/cm at 980 nm. On the other hand, the pulsed LIDT scales with the square root of the laser wavelength and the square root of the pulse duration, resulting in an adjusted value of 55 J/cm2 for a 1 µs pulse at 980 nm. The pulsed LIDT of the optic is significantly greater than the energy density of the laser pulse, so individual pulses will not damage the wave plate. However, the large average linear power density of the laser system may cause thermal damage to the optic, much like a high-power CW beam.
| Posted Comments: | |
Jiahui Huang
(posted 2024-09-12 15:55:59.25) Hello Thorlabs,
Can you provide the information of GDD as a function of wavelength (400-1000nm) for SAHWP05M-700?
Thank you! jpolaris
(posted 2024-09-12 01:45:30.0) Thank you for contacting Thorlabs. Requests for additional data and specifications can be made by emailing us at techsupport@thorlabs.com. I have reached out to you directly with the GDD data for SAHWP05M-700 that you have requested. Xukun Feng
(posted 2024-09-11 21:10:04.817) 能否提供该产品对应波长的透过率与反射率 cdolbashian
(posted 2024-09-17 03:47:55.0) Thank you for reaching out to us with this inquiry. The question you asked is as follows: "Can you provide the transmittance and reflectance of the corresponding wavelength of this product?". The transmission and reflectance of these waveplates can be found by clicking the blue "i" next to the product in the specifications table. Boris Považay
(posted 2024-08-28 14:44:22.83) Do you have more information on the design (e.g. total glass thickness, angular dependence) or even an optical design file (also blackbox) available?
Best regards! jdelia
(posted 2024-09-05 09:25:17.0) Thank you for contacting Thorlabs. The total glass thickness is about 2 mm. Angular dependence can be found in the raw data we offer, which can be accessed by clicking the blue "i" icon under "Specifications" on the product family page. We unfortunately do not have an optical design file at this time. We have reached out to you directly via email to discuss your application and whether these items would work for your experiment. Kimi Huang
(posted 2024-03-05 12:52:26.08) hi Sir,
What is the lead time of this part if I would like to order 5 pcs cdolbashian
(posted 2024-03-05 03:31:01.0) Thank you for reaching out to us with this inquiry. At the time of posting, I have contacted you regarding the lead time for this item. user
(posted 2022-11-16 13:13:53.873) Hello Thorlabs! How robust is retardation against temperature change (around room temperature)? cdolbashian
(posted 2023-01-23 12:18:28.0) Thank you for reaching out to us with this inquiry. Unfortunately we do not have temperature-dependent phase data for this component for small fluctuations about room temp. Due to the materials used and their thermal properties, we do not expect a significant change in performance while within room temperature. Yumin Sim
(posted 2022-11-01 11:15:40.727) Dear Thorlabs,
Currently, using SAQWP05M-700, PL signal imaging is performed along the circularly polar. direction.
However, when the Quarter Wave Plate (QWP) is rotated, the physical position of the PL signal in the CCD detector is slightly changed. It's probably because of the beam deviation of QWP, right? To explain in more detail, it is inferred that if the QWP is rotated, the deviated beam pass also rotates. Please tell us exactly the cause or principle of beam deviation of QWP. I want to know exactly how beam deviation occurs. I'm asking because I couldn't find the exact information about the beam deviation of QWP.
And, if there is a way to reduce or eliminate the beam deviation effect, please let me know.
Lastly, is it possible to order and purchase the customized products with minimal beam deviation? cdolbashian
(posted 2022-11-08 01:45:44.0) Thank you for reaching out to us Yumin. Depending on the degree of deviation, this might be attributed to the beam deviation spec. This can be attributed to the thick stack of birefringent materials. Any small wedge in the stack causing rotational asymmetry will yield some sort of deviation. I have reached out to you regarding some more specifics. Simon Neves
(posted 2022-09-16 22:35:02.39) PS to my previous comment: I obviously meant "surface coating", not quoting. Apologies. Simon Neves
(posted 2022-09-16 22:27:46.803) I see these plate are quite lossy (90% transmission) and I assume this is due to the absence of optical quoting, compared to single-wave length wave plates. I was wondering if it was possible to ask for a surface quoting, to get more transmission in specific wavelengths. In our case, we would require a high transmission around 1550nm (+/- 10nm), and still keep a pretty high transmission at 775nm (at least 90%). Could you tell me what solution you could propose?
Thank you very much!
Simon cdolbashian
(posted 2022-10-04 02:56:41.0) Thank you for reaching out to us with this inquiry! These plates already have a dielectric AR coating on them coating both sides. Due to the construction of these achromatic waveplates, there are many surfaces which need to be traversed in the material accumulating this loss. For future custom requests please feel free to reach out to our Solutions Team (Techsales@thorlabs.com) user
(posted 2020-02-20 06:32:26.79) We have seen non-negligible fast axis deviation at two wavelengths, 665nm and 1120nm on the superachromatic waveplate SAQWP05M-1700, seems larger than the calculated values. Can it be improved? nbayconich
(posted 2020-02-27 09:31:54.0) Thank you for contacting Thorlabs. I will reach out to you directly to discuss your application and the values you had measured. David Vojna
(posted 2019-11-11 10:30:04.203) Dear Sir/Madam
I would like to ask whether you could share with me the retardance of the SAHWP05M-1700 wave plate between 500 to 600 nm?
Thank you.
Best
David Vojna YLohia
(posted 2019-11-11 11:18:41.0) Hello David, thank you for contacting Thorlabs. The retardance performance in this extended range is given in the raw data file that is brought up when you click the blue "info" icon next to the part number and going over to the "Retardance" tab on the pop-up. Here is a direct download link: https://www.thorlabs.com/images/popupimages/SAHWP05M-1700.xlsx user
(posted 2019-08-22 11:25:36.553) Do you have 1 inch version of SAHWP05M-1700 and SAQWP05M-1700? YLohia
(posted 2019-08-22 05:14:40.0) Hello, thank you for contacting Thorlabs. Custom items can be requested by emailing techsupport@thorlabs.com. I have reached out to you directly to discuss the possibility of offering this. blanchet valerie
(posted 2019-03-15 11:43:18.267) dear thorlabs.
Just received a SAQWP05M-700. this quarter waveplate is mounted in autocollimation of a 400nm vertically polarized beam. The image of the focused beam (focal length of 300 mm) is recorded as function of the rotation of the quarter wave plate.The position of the focused beam rotates by 70microns with the rotation of the quarter waveplate. What is the wedge tolerance of this quarter waveplate ? nbayconich
(posted 2019-03-19 03:45:07.0) Thank you for contacting Thorlabs. We do not specify a wedge tolerance however we do specify the beam deviation for these superachromatic waveplates to be <3arcminutes. Given the distance traveled this displacement should be within specification of the superachromatic waveplates.
Our achromatic waveplates will have a much tighter beam deviation tolerance of <15 arseconds, which may be an alternative option. user
(posted 2018-07-09 22:33:07.93) Hi,
I would like to use these waveplates for a 1kHz 100 fsec pulses centered around 400 nm. Would it be fine or would it damage the waveplates ? YLohia
(posted 2018-07-10 04:46:05.0) Hello, unfortunately, we have not performed damage threshold testing on these waveplates with femtosecond sources. We do, however, have the following information: 1500 W/cm (CW, 1064 nm, Ø0.091 mm) and 0.5 J/cm² (1064 nm, 10 ns, 10 Hz, Ø1 mm). It is highly recommended to be at least within these power levels as a guideline. Please note that the test data for CW or ns regime cannot be directly scaled to provide useful information about the fs regime. user
(posted 2018-05-10 22:03:33.877) Wow! wavelength dependency on retardance is 0.5 waves ±0.03% or better if I read correctly the graph. I has been looking for that. Other company provides only 0.5 waves +/-0.01 waves.... Again, My reading correct? YLohia
(posted 2018-05-14 08:51:16.0) Hello, yes, those plots show the theoretical deviations in retardation as a function of wavelength, same as provided by other companies. The actual performance can differ slightly from these specs. Since you did not leave your contact info, please reach out to us via email if you would like to discuss this further. simon.neves
(posted 2018-04-30 09:07:35.567) Hello,
I see that the retardance is said to be around 0.5 or 0.25 wave-length. I assume that the SAWP are zero-order then. Can you confirm that assumption ?
Thank you ! YLohia
(posted 2018-04-30 09:18:07.0) Due to the nature of the Pancharatnam-type design, these superachromatic waveplates are not true-zero order. e0167142
(posted 2018-04-03 20:20:32.763) Hello, I would like to know your product SAHWP05M-1700's temperature dependence. For example if the temperature of environment fluctuates between 22 and 25 degree C, how would it affect the performance of your product. And is this true zero order?
Thank you! YLohia
(posted 2018-04-04 09:08:20.0) Hello, thank you for contacting Thorlabs. While we don't currently have a temperature dependence plot for SAHWP05M-1700, we do not expect a noticeable difference in performance with environmental temperature fluctuations between 22 and 25 degrees C since this is made of quartz and MgF2, which do not have a significant temperature dependence within that range. Due to the nature of the Pancharatnam-type design, these superachromatic waveplates are not true-zero order. dvalovcin
(posted 2017-12-29 15:05:13.973) What's the expected tolerance on the surface normal angle relative to the housing? The back reflection moves significantly when rotating the optic through 360°. tfrisch
(posted 2018-01-16 02:06:20.0) Hello, thank you for contacting Thorlabs. While we don't have a formal tolerance on the parallelism of the optic face and the housing face, the waveplate is held against the inside of the housing by a retaining ring. I will reach out to you directly about the angle of the precession you are seeing and what impact the rotation stage might have as well. asherw
(posted 2017-08-02 11:48:44.203) I'd like to know the approximate thickness of this waveplate outside of the mount (total crystal layer thickness) and find out if it could be ordered unmounted.
Thanks,
Asher tfrisch
(posted 2017-08-16 02:22:43.0) Hello, thank you for contacting Thorlabs. There will be some variation on the thickness of the optic assembly, I'll reach out to you with more details on the dimensions and a quote. leonard.monniello
(posted 2016-09-05 11:04:46.083) What is the polarization conservation of the fast axis of the SAQWP05M-700 ? Right now, in between two polarizers with up to 10^5 of polarization ration, I got 10^3 when adding the QWP, at the very best. Thanks for your help tfrisch
(posted 2016-09-08 08:48:20.0) Hello, thank you for contacting Thorlabs. I have contacted you asking for more details on the experimental setup. florian.enderli
(posted 2016-04-26 12:30:15.953) Can the superchromatic wave plates be used under ultra high vacuum conditions? This would depend on whether the glue used to cement the individual quartz and magnesium fluoride plates does outgas under these conditions or not. Thanks for your help. besembeson
(posted 2016-04-26 10:23:38.0) Response from Bweh at Thorlabs USA: The glue that we use is a low out-gassing epoxy so depending on your vacuum level, it may be suitable. I will follow up with you. neil.troy
(posted 2014-12-17 14:06:36.893) Can you provide the measured reflection across the full bandwidth? Or 100%-Transmission can be assumed to be reflected? myanakas
(posted 2015-01-12 04:07:50.0) Response from Mike at Thorlabs: Thank you for your feedback. The reflectance should not be assumed to be 100%-Transmission. This data can be found in the blue info icons located in the gray tables immediately above the ordering area. |
Choosing a Wave Plate
Thorlabs offers achromatic, superachromatic, zero-order (both unmounted wave plates and mounted wave plates), low-order, and multi-order wave plates (single wavelength and dual wavelength) with either λ/4 or λ/2 phase shift.
Achromatic Wave Plates provide phase retardance that is relatively independent of wavelength over a wide spectral range, and Superachromatic Wave Plates provide phase retardance almost entirely independent of wavelength over a much wider range than achromatic wave plates. In contrast, zero-order and multi-order wave plates provide a phase shift that is strongly wavelength dependent. Our achromatic wave plates are available with four operating ranges: 260 - 410 nm, 350 - 850 nm, 400 - 800 nm, 690 - 1200 nm, and 1100 - 2000 nm. Additionally, we offer superachromatic wave plates for the 310 - 1100 nm and 600 - 2700 nm ranges.
| Round Zero-Order Wave Plate Comparison | ||
|---|---|---|
| Material | Quartz | LCP |
| Sizes | Ø1/2" and Ø1" | Ø1/2", Ø1", and Ø2" |
| Mounted Versions Available | Yes | Yes |
| Retardances Available | 1/4 λ and 1/2 λ | 1/4 λ and 1/2 λ |
| Retardance Accuracy | <λ/300 | <λ/100 |
| Surface Quality | 20-10 Scratch-Dig | 60-40 Scratch-Dig |
| Coating | V Coat | Broadband AR |
| Coating Reflectance (per Surface) |
0.25% | <1.0% Average Over Specified Coating Range |
Zero-order wave plates are designed such that the phase shift created is exactly one quarter or one half of a wave. They offer substantially lower dependence on temperature and wavelength than multi-order wave plates. Our Zero-Order Quartz Half-Wave and Quarter-Wave Plates are composed of two wave plates stacked together with the fast axis of one aligned to the slow axis of the other to achieve zero-order performance. Thorlabs' zero-order wave plates are available for a number of discrete wavelengths ranging from 266 nm to 2020 nm. Our Polymer Zero-Order Half-Wave and Quarter-Wave Plates consist of a thin layer of liquid crystal polymer retarding material sandwiched between two glass plates and are available at discrete wavelengths between 405 nm and 2700 nm. Our quartz zero-order wave plates provide better retardance accuracy and lower reflectance (see table), while our LCP zero-order wave plates produce a smaller decrease in retardance at larger AOIs. In addition, Thorlabs also offers unmounted true Zero-Order Telecom Wave Plates for WDM applications.
MIR Wave Plates are made from a single piece of high-quality magnesium fluoride and provide either quarter-wave or half-wave retardance at 2.5 µm, 2.713 µm, 2.94 µm, 3.5 µm, 4.0 µm, 4.5 µm, or 5.3 µm. Light passing through these MIR wave plates will undergo a low number of full or partial wavelength shifts (also referred to as the order, or m) in addition to the fractional design retardance. This differs from true zero-order and multi-order wave plates which undergo no shift or a high number of shifts, respectively. The low-order design maintains near to true zero-order performance, making it a good alternative to true zero-order wave plates. The single magnesium fluoride substrate is also thinner compared to a zero-order design, which combines two multi-order wave plates, making our low-order retarders well suited for applications that are sensitive to dispersion.
Multi-Order Wave Plates are made such that the retardance of a light path will undergo a certain number of full wavelength shifts (also referred to as the order, or m) in addition to the fractional design retardance. Compared to their zero-order counterparts, the retardance of multi-order wave plates is more sensitive to wavelength and temperature changes. Multi-order wave plates are, however, a more economical solution for many applications where increased sensitivities are not an issue. Our multi-order wave plates are available for a number of discrete wavelengths ranging from 405 nm to 1550 nm. Thorlabs also offers Dual-Wavelength Multi-Order Wave Plates designed for use at both 532 nm and 1064 nm.
In addition to these options, Thorlabs also has the ability to design and manufacture custom wave plates for both OEM sales and individual low quantity orders. Our technical staff is able to help with all phases of your request: quoting, sales, and planning and manufacturing support. If you have a custom request or a question about our capabilities, please contact Tech Support to start a discussion.
 Products Home
Products Home













 Zoom
Zoom

 Superachromatic Wave Plates
Superachromatic Wave Plates